对等向强化，文献[1-2]定性给出下式：

本文给出了稳定材料比例加载条件下后续屈服当硬化指数f′≈n≠E=tanα时，式（1）数学方程的具体形式并几何描述强化曲面.

1   单向拉伸强化

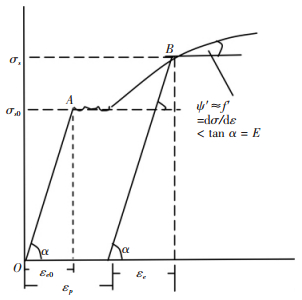
如图 1，A点对应弹性应变的应力球分量为：

图  1  单向拉伸初始与后继屈服

下载: 全尺寸图片 幻灯片

式（2）中：σ₂=σ₃=0；σ_s0为初始屈服点.

B点为σ₁=σ_s，σ_s＞σ_s0，

将体积改变定律^[3-4]代入式（3）得：

（5）、式（6）表明单向拉伸后续屈服极限σ_s的增加伴随着ε_m与σ_m的增加.在等倾空间ε_m的变化取决于屈服柱面轴线oH与原点O的距离，如图 2.称该距离为球应力矢量^[4-5]，即ON₀² =3σ² _m0，为此σ_m的增加意味着材料的硬化使后续屈服柱面的垂直截面沿H离原点越来越远.由于多数强化材料后续屈服发生总伴随一定递增的平均应力，故平均应力增加幅度决定了初始屈服轨迹与后续屈服轨迹在Mises柱面上沿H移动的垂直距离（若存在异号主应力，且σ_m=σ₁+σ₂+σ₃=0，则π平面经过原点，σ_m与强化无关）；而屈服半径的增加决定于偏差应力增加的幅度或后续硬化指数f′.

图  2  屈服柱面

下载: 全尺寸图片 幻灯片

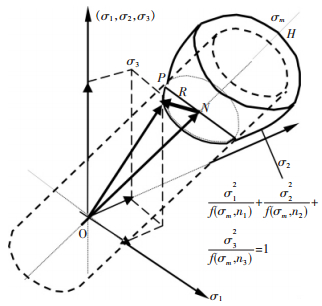
2   等斜率强化曲面方程

如图 2，设复杂应力状态在等倾空间上的位置为P₀（σ₁₀，σ₂₀，σ₃₀），且该点发生初始屈服.于是为处于屈服柱面上的应力矢量，为初始屈服半径，H为初始屈服柱面轴线.上述各量为^[5]：

令初始屈服时应力矢量与H夹角为α，则

需指出，对比例加载，当应力矢量在Mises空间的方向不改变，即后续屈服发生时硬化指数为常数或f′=tgα时，则初始屈服柱面内各点均处于弹性状态，故图 2中以H为轴心，矢量绕H旋转得到的圆锥面Oaa′为一弹性锥面，且H满足

上式表明在H上的点满足σ₁=σ₂=σ₃.

对于延长线上任一点P（σ₁=σ₂=σ₃），如图 3, 到原点与H的距离分别为：

sinα=PN/OP，将式（8）、式（9）代入得：

展开、整理并注意到^[6]sec²α=1+tg²α，得：

式（10）即为图 2弹性锥面Oaa′的曲面方程.对比例加载，且f′=tgα为常数，将式（7）代入式（10）得

式（11）中σ₁₀，σ₂₀，σ₃₀为初始屈服应力矢量端点的坐标，α为弹性硬化锥角.式（10）、式（11）为主应力空间以坐标σ₁，σ₂，σ₃为变量的弹性二次锥面方程的不同形式.圆锥轴线σ_m等倾54°44′.

欲检验式（10）与式（11）的可靠程度，将弹性锥面边界点P₀（σ₁₀，σ₂₀，σ₃₀）代入式（11）得：

展开并整理得：

即为初始Mises屈服准则.说明式（11）无误.

设后续屈服某瞬时强化材料的屈服应力为σ_s，应力矢量为，图 3中P点坐标为：P（σ₁₀+dσ₁，σ₂₀+ dσ₂，σ₃₀ +dσ₃）=P（σ₁，σ₂，σ₃），则后续屈服条件为：

由高等数学（x₁-x₂）²+（x₂-x₃）²+（x₃-x₁）²=3R²可知式（13）是半径为，等倾54°44′的圆柱面方程.的端点落在柱面P点上（如图 3），且

图  3  强化指数为常数tanα的硬化曲面

下载: 全尺寸图片 幻灯片

注意到柱面与锥面方程联立表示一条空间曲线即柱面与锥面的交线，于是联立有：

式（14）即为等向强化后续屈服轨迹在主应力空间上的曲线方程^[7]，其在π平面上的投影为一系列半径线性递增同心圆.求解式（14）得：

式（15）仅是式（14）的不同形式.因此比例加载下强化曲面则为图中以初始屈服轨迹aa′为底、由这些同心圆组成的圆台aa′bb′的侧表面^[7]如图 3.其物理意义在于：随着强化进行，尽管屈服半径和轨迹离原点距离同时增加，但二者比值不变，且半径增大直至材料失稳破断为止.其数学方程为下式，

3   变斜率强化曲面方程

然而绝大多数材料初始屈服后强化斜率发生变化，且dσ/dε＜σ/ε（切线斜率小于割线斜率）即

此时单向拉伸曲线发生弯曲，后续屈服有

将其代入到σ=f（ε）中，后求导有^[8]：

该式变形为：

这是因为，代入式（15）并注意此时tanα= 已是逐渐减小的变量，于是有：

把f′表达式代入到式（10）经整理得：

式（19）为标准球面方程^[9].该式表明强化球面的半径

假定材料为非线性强化且按幂率强化^[10-11]

式（20）中K是强度系数即产生塑性真应变为1时的真应力值；n是硬化指数；注意到拉伸塑性失稳时^[12-13]

如三轴强化系数满足上式并相等, 强化曲面为球面^[9]

实际上尽管屈服柱面的同一截面上具有相同的静水压力，但多数情况下因材料性能差异致使三轴在比例加载条件下硬化指数也不能完全一致，即：n₁≠ n₂≠n₃≠n，于是硬化曲面的标准方程变为

此时硬化曲面为标准椭球面^[9]，如图 4.

图  4  变斜率等向化曲面几何描述及数学方程

下载: 全尺寸图片 幻灯片

曲面具有如下性质：

如果两向拉伸硬化指数相等，即f′₁=n₁=f′₂=n₂，则强化椭球面变成回转椭球面，强化状态对称于oσ₃轴.

如果三向拉伸的强化系数都相等，即n₁=n₂=n₃= n=f′，则强化曲面变成圆球面，此时球面强化方程为式（19）及式（22）.

如果两向拉伸且强化系数都不等，即n₁≠n₂，σ₃=0，则强化曲面轨迹变成二维椭圆^[9]，如图 5.此时强化方程满足：

图  5  后续随动等向强化σ₃=const的轨迹

下载: 全尺寸图片 幻灯片

还应特别指出：对无包辛格效应材料，在应力状态为负时，相应硬化曲面图形与图 3、图 4、图 5沿OH负向相对o点对称.以上讨论仅适合严格按Drucker公设的稳定材料.

4   结论

（1） 随动线性强化的曲面为圆台面，后续轨迹为一系列同心圆，特点为比例加载条件下f′=n=tanα不变.方程满足式（15）、式(16).

（2） 条件下比例加载随动强化为非线性强化，三轴强化系数不等时强化曲面为椭球面，二维时为椭圆.方程满足式（23）、式(24).

（3） 满足幂率方程的强化材料，若三轴强化系数相同即f′=n₁=n₂=n₃时，比例加载的随动强化曲面为球面.方程满足式（22）.

（4） 以上结论仅适合满足Drucker公设的稳定材料.