On the thermal decomposition kinetics of graphite fluoride based on non-isothermal thermogravimetry
-
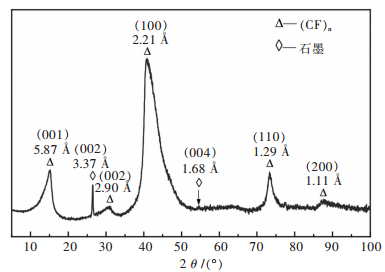
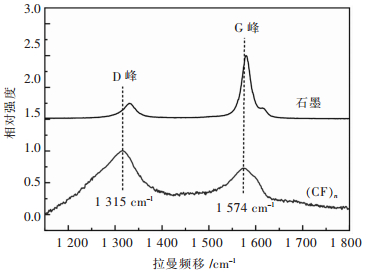
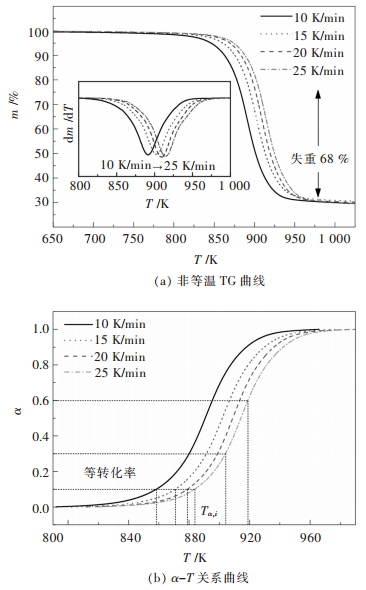
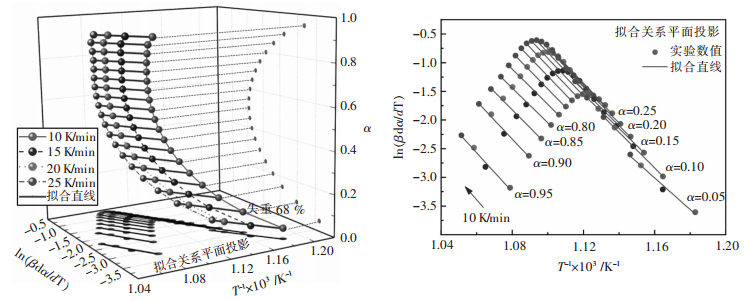
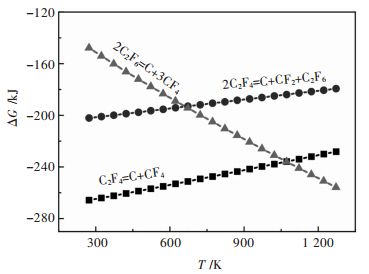
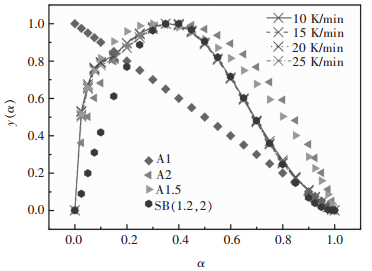
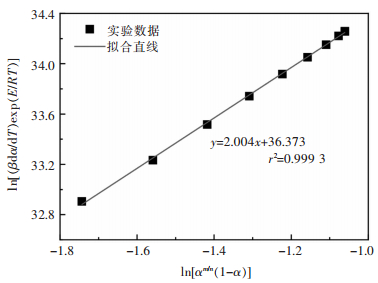
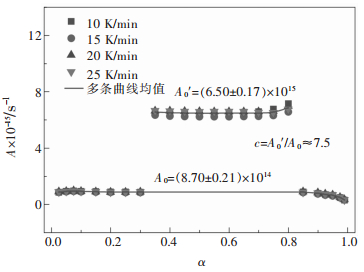
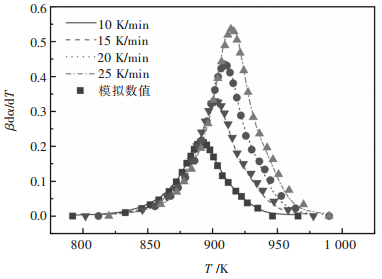
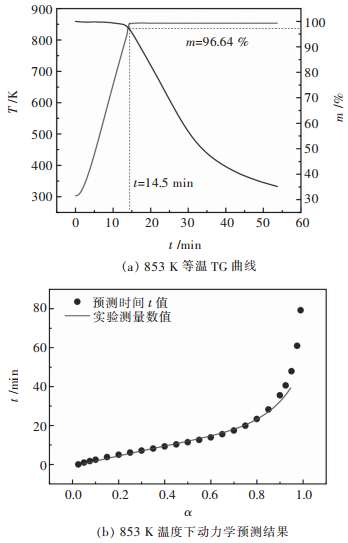
摘要: 针对氟化石墨热分解动力学机理不确定和动力学预测信息不足的问题，通过测量多组非等温热重曲线，并利用无模型动力学方法分析（CF）n热分解反应动力学机理.热重曲线显示（CF）n热分解经历一步失重，产生的平均气相成分为CF2.95.动力学分析结果表明机理函数随转化率依次变化：α < 0.1，机理函数为JMA方程f（α）=1.5（1-α）[-ln（1-α）]1/3；0.15 < α < 0.3，机理函数为二维Avrami-Erofeyev方程f（α）=2（1-α）[-ln（1-α）]1/2；0.3 < α < 0.8，机理函数为Šesták-Berggren方程f（α）=7.5α1.2·（1-α）2；0.85 < α，机理函数为一维Avrami-Erofeyev方程f（α）=（1-α）.推荐的动力学预测参量活化能为264.23±7.82 kJ/mol，指前因子为（8.70±0.21）×1014/s.另外，动力学机理反映出（CF）n分解过程存在碳核的链生长以及与分支链的相互作用的特征，这可能是反应产物形成非晶态结构碳的重要因素.Abstract: Aiming at the problem of uncertain mechanism of kinetics of thermal decomposition of fluorinated graphite and insufficient information of kinetic prediction, the dynamic mechanism of (CF)n thermal decomposition reaction was analyzed by measuring multiple sets of non-isothermal thermogravimetric curves and using model-free kinetics. The thermogravimetric curve shows that (CF)n thermal decomposition undergoes one step of weight loss, resulting in an average gas phase composition of CF2.95. The results of kinetic analysis show that the mechanism function changes with the conversion rate: α < 0.1, the mechanism function is JMA equation f(α)=1.5(1-α)[-ln(1-α)]1/3; 0.15 < α < 0.3, the mechanism function is two-dimensional Avrami-Erofeyev equation f(α)=2(1-α)[-ln(1-α)]1/2;0.3 < α < 0.8, the mechanism function is Šesták-Berggren equation f(α)=7.5α1.2(1-α)2; 0.85 < α, the mechanism function is the one-dimensional Avrami-Erofeyev equation f(α)=(1-α). The recommended kinetic prediction parameter activation energy is 264.23±7.82 kJ/mol, and the pre-exponential factor is (8.70±0.21)×1014/s. In addition, the kinetic mechanism reflects the existence of carbon chain chain growth and interaction with the branching chain in the (CF)n decomposition process, which may be an important factor in the formation of amorphous structural carbon in the reaction product.
-
Keywords:
- graphite fluoride /
- kinetic mechanism /
- CF4 /
- model-free kinetics method /
- solid phase decomposition
-
0 引言
镁合金是一种新型的金属材料，它具备高强度、高刚度、强电磁屏蔽能力和耐辐射能力.具有等轴晶的超细晶镁合金广泛应用于汽车、电子、航空航天和国防等领域.许多国家将其视为21 世纪重要的战略资源，并提出了许多研究和发展计划.在这种背景下，研究超细晶镁合金的细化机制和生产过程是十分重要的.为了使镁合金在工业领域有良好的应用，提出了许多提高镁合金力学性能的方法.细化晶粒和控制结构是一种有效地提高金属材料性能的方法.细化晶粒可以提高镁合金在室温下的强度、延展性和超塑性成形能力.
晶粒细化的方法有很多，如挤压、锻造、轧制及其之后的再结晶退火过程.等通道转角挤压（ECAP）作为一种成形技术，能够细化材料组织、改善性能，现已在塑性成形领域得到广泛应用.等通道转角挤压原理如图 1 所示.等通道转角挤压是将工件压入模具以实现大剪切变形量的工艺.在变形过程中，工件从模具通道强行挤出，材料发生剪切变形，获得大的剪切应变，从而细化晶粒.等通道弯角挤压模具是由具有相同横截面积的竖直通道和水平通道相交接而成，并形成两个交接角，即模具拐角Φ 和模具圆心角Ψ [1-2].利用该方法可制备出亚微米级超细晶显微结构的铝[3-6]，铜[7-8]，镁[9-11]，钛[13，14]等金属或合金.不同的工艺参数对经ECAP 变形后的镁合金的组织和性能有不同的影响[15].本文选取了模具拐角、模具圆心角、摩擦力和挤压道次4 个工艺参数进行模拟，并对模拟结果进行了分析.
1 模拟分析
在模拟过程中，挤压件材料为镁合金AZ31，尺寸为12 mm×12 mm×60 mm，网格划分数为10 000，假设挤压件为刚塑性，其应力应变关系为σ =C$\varepsilon \overset{m-n}{\mathop{\varepsilon }}\,$ ，其中C=205 MPa，应变硬化参数m=0.114，n=0.044，挤压速度是10 mm/s.由于镁合金在室温下塑性很差，模拟时设模拟温度为250 ℃.
1.1 模具拐角的影响
对于模具拐角，主要研究90°、100°、110°和120°4 种情况，利用DEFORM 软件对上述不同模具拐角挤压过程进行分析，获得挤压件的等效应力分布、等效应变分布和加载载荷曲线，通过对等效应力和等效应变以及加载载荷的分析，确定合理的模具参数.
从图 2 分析不同模具拐角下挤压件的等效应变的分布可以发现，90°拐角时挤压件的最大等效应变较大且挤压件上的等效应变分布与等效应力的分布情况基本相同.由此可见模具拐角为90°比其他3 种模具拐角的变形范围更广，变形量更大，挤压件在流经模具拐角处应变最大，且靠近内拐角处获得的等效应变最高，而内拐角处获得的最高等效应变正是挤压件所需要获得的主要变形，是挤压件通过ECAP 工艺达到细化晶粒目的的主要方式.从图 3 看出挤压载荷随着模具拐角的增大，挤压载荷逐渐减小.在实验设备均能满足上述要求的情况下，在模具工艺设计过程中应结合能源消耗综合考虑.
1.2 模具圆心角的影响
图 4给出了模具拐角为90°时，不同模具圆心角所对应的工件截面上等效应变分布和有限元模拟等通道转角变形过程中试样形状的变化.从模拟结果可以看出，试样每个部分的变形并不均匀.试样的头部和尾部的变形很小.试样中部的变形相对均匀.试样横截面中间区域的变形要比截面上下部位剧烈，这将对变形的均匀性产生不利的影响.
表 1给出了最大挤压载荷、最大等效应力和最大等效应变之间的关系.随模具圆心角的增大，等效应力有所下降.调整模具圆心角的大小对模具拐角处等效应变的分布有重要影响.但对内拐角处的影响较小.因此当模具拐角处应力较为集中，在模具设计过程中应设计一定倒角，从而避免模具拐角处应力过于集中.挤压件在等效应变的分布大致情况与等效应力相似.模具圆心角越小，等效应变就越大.等效应变从外拐角向到内拐角逐渐增大.模具圆心角Ψ 越大，累积总应变越小.在模具设计中不仅要考虑挤压件变形量的累积，还要考虑到挤压件变形的均匀性和模具圆心角的合理选择，随着圆心角的增大，最大挤压载荷逐渐减小.所以挤压时模具圆心角不宜过小.
表 1 模具圆心角不同时，最大挤压载荷、最大等效应力和最大等效应变的关系
因此，综合考虑挤压过程的等效应力演化规律，挤压后工件的应变累积规律以及载荷变化规律，必须慎重选择模具圆心角.为了获得变形分布更好、应变更高的试样，模具圆心角取值范围一般应等于或略大于30°.
1.3 摩擦的影响
摩擦对等通道转角挤压变形具有重要影响.分析不同摩擦条件对挤压过程变形机理的影响，获得挤压件变形分布规律，了解和掌握变形载荷，进而为提高模具寿命提供理论指导.图 5 是不同摩擦因子时，工件的网格变化情况.从模拟结果可以看出，不同摩擦因子时，挤压件的变形情况相似，但变形大小各不相同，且挤压件整体的变形的均匀性也不相同.通过模拟分析，从表 2 可以看出，随着摩擦因子的增大，等效应力增大，但增大趋势不甚明显.因此，摩擦对于改善挤压件的受力大小不明显.从图 5 看出，摩擦因子对等效应变的影响也不是很大.但摩擦因子的大小对挤压载荷的影响十分明显，随着摩擦因子的增加，挤压载荷显著增加，反之亦然.因此，从降低挤压载荷和提高模具寿命角度而言，应尽可能地减小摩擦，即挤压过程中应采取较好的润滑措施，可有效降低挤压载荷，提高模具使用寿命.
表 2 不同摩擦因子时，最大等效应变、最大等效应力与最大载荷的关系
1.4 挤压道次的影响
通过对上述模拟结果分析得出，选择合理的模具几何形状以及工艺参数可提高挤压件的变形分布均匀程度与应变累积量，但是单道次ECAP 所获得的挤压件累积变形量较小且变形分布很不均匀.为了获得变形比较均匀的挤压件，挤压件需要累积较大的应变量.因此，多道次挤压有利于累积工件的应变量.本文分别对挤压件进行了4 个道次的模拟，并分析了等效应力和等效应变以及载荷曲线，有利于挤压道次的合理选择.
表 3给出了当模具拐角为90°，挤压道次不同时，最大挤压载荷、最大等效应力和最大等效应变的关系.从表 3 中可以看出挤压4 道次后，通过变形的累积，等效应变随挤压道次的增加而增加.挤压载荷也随着挤压道次的增加而增加，但是增加的幅度并不明显.
表 3 不同挤压道次时，最大挤压载荷、最大等效应力和最大等效应变的关系
图 6给出了挤压道次分别为1、2、3 和4 道次时，等效应变沿横截面高度的分布曲线.挤压件经过四道次后的应变分布并不均匀.从上表面到下表面，等效应变呈现先增加后降低的趋势.变形主要集中在挤压件的中部，上下表面的变形较小.从图 7 还可以看出从第1 道次到第4 道次挤压件的应变逐渐累积，应变逐渐增大.重复挤压以后，挤压件的晶粒受到了不同水平的细化，挤压载荷也相应提高.综上所述，挤压件具有最佳性能，即指获得一定的应变的同时应力不会太高.
2 ECAP 对镁合金力学性能的影响
2.1 AZ31 镁合金经ECAP 变形微观组织的演变
通过DEFORM 模拟了AZ31 镁合金ECAP 变形晶粒网格的变化，通过分析可以看出，ECAP 可以使 AZ31 镁合金晶粒得到细化.而许多科研人员也通过实验证实了这一观点.冯晓明和艾桃桃[16]在673 K 下，对镁合金进行ECAP 变形，并观察了不同道次挤压下材料内部显微组织的变化.未经ECAP 变形的原始晶粒尺寸约为120 μm，经过一道次挤压后，晶粒尺寸降低到18 μm 左右，晶粒明显得到细化.随着挤压次数的增加，晶粒进一步得到细化.通过图 7 可以看出温度对晶粒尺寸的影响是十分显著的.随着温度的增加，晶粒粗化，晶粒尺寸增加.因此，在满足工艺要求的情况下，应尽量选择较低的温度.
2.2 AZ31 镁合金经ECAP 变形力学性能的变化
2.2.1 屈服强度和抗拉强度
AZ31 镁合金经ECAP 后随着挤压道次的变化，力学性能也发生相应的变化.未挤压时材料的屈服强度为182.5 MPa，经过1、2 道次挤压后合金的屈服强度略有降低，分别降低了5.47 %和8.22 %.出现与 Hall-Petch 公式不符合的现象，这是因为屈服强度受到晶粒尺寸和织构的影响.ECAP 过程中，4 道次挤压前织构的最大极密度不是很大，织构变化并不明显且形成的都是软取向织构，因此屈服强度的提高主要是晶粒细化的结果；而传统挤压不同，经过温热挤压后，晶粒极大细化且形成方向平行于挤压方向的{0001}基面纤维织构，由于{0001}基面纤维织构是硬取向织构，室温拉伸时基面很难发生滑移，因此在一定程度上提高了合金的屈服强度，晶粒的细化作用加上织构的强化作用是传统挤压材料的屈服强度高于ECAP态材料的原因.经ECAP 4 道次变形以后，晶粒更加细小，织构也得到加强，屈服强度和抗拉强度得到了很大提高.因而随着道次的增加，合金的微观组织的均匀性增加，织构也逐渐得到加强，屈服强度开始随着晶粒的减小而增加，2、3、4 道次屈服强度也逐渐提高，四道次后屈服强度能达到215.3 MPa，由此可以看出AZ31 镁合金经4 道次ECAP 变形后，屈服强度得到明显提高.四道次后抗拉强度达到311.2 MPa，也得到相应提高.因此，四道次后可以明显改善AZ31镁合金的强度.
2.2.2 宏观硬度
AZ31 镁合金经ECAP 变形后，宏观硬度有显著提高.试样随挤压道次增加，宏观硬度值有显著增加.刘自乾等[17]通过实验分析了不同道次对材料力学性能的影响.如图 8 所示.未经过ECAP 变形工件的原始硬度为43.69 MPa，一道次挤压后材料的硬度63.57 MPa，随道次的增加，硬度也持续增长，3 道次达到饱和，硬度值达到90.81 MPa.4 道次后材料的硬度略有降低并在随后的道次中材料的硬度基本保持一致，没有发生显著的变化.这是由于随着晶粒细化，晶界处位错塞积减少，位错储能降低，同时，晶粒细化，动态回复能力提高.动态回复软化与加工硬化趋于平衡．硬度上升缓慢：4 道次后组织中晶粒基本是细小的等轴晶，晶粒间变形协调更好，整体位错减少，动态回复带来软化效应基本与加工硬化基本趋于平衡．在随后道次，组织微观形貌基本不变.虽然晶粒尺寸随变形的继续有所减低，但是宏观硬度值基本不变.
2.3 ECAP 变形AZ31 镁合金晶粒尺寸与等效应变的关系
晶粒细化对材料的性能有显著地影响，根据 Hall-Petch 公式可知，随着晶粒尺寸d 减小，材料硬度和强度都有所上升.因此如何简单有效地分析晶粒细化程度便显得至关重要.通过多道次ECAP 工艺，可以使材料内部累积均匀的应变，使晶粒得到细化.然而，至今为止对晶粒细化与累积等效应变分布及均匀程度之间的关系的研究并不多，因此，研究晶粒细化与累积等效应变分布及其均匀程度之间的关系十分必要.
在前期工作的大量实验与理论推导结果的基础上，通过高斯公式建立和推导ECAP 累积等效应变、挤压件组织结构细化后主要变形区平均晶粒度与对应挤压道次下变形材料的力学性能指标之间的定量关系，通过应变的累积，预测超细晶镁合金ECAP 变形后可能达到的平均晶粒度和力学性能.
针对材料晶粒平均几何尺寸大于100 nm 的超细晶材料，其晶粒度对强度的影响可用Hall-Petch（霍尔-佩奇）公式描述为：
(1) 式（1）中，d 为晶粒平均尺寸，n 随d 有一定变化，典型值一般为0.5，σs为材料屈服强度，通常可用显微硬度Hv来代替，σi为晶粒中位错运动所需的应力，Ky为材料常数.上述公式只是晶粒度与力学性能之间的关系，而采用高斯公式推导可得出主要变形区平均等效应变对应的变形材料的力学性能的新方法：
(2) 式（2）中，ε軈是挤压件等效应变，K0是当变形材料达到一定情况的强度和强韧性对应的关键应变，K1是修正的因子，K1∈（0，1），K0和K2是当材料达到一定状态时独立于关键应变和力学性能2 个参数，K2 /K1是一定状态时材料的力学性能值.因此，将式（1）代入式（2），可以建立表征ECAP 累积等效应变和细化晶粒度的新方法：
(3) AZ31 镁合金在2、3、4 道次变形后材料的晶粒尺寸与屈服强度的关系如图 9.经计算得符合Hall- Petch 公式，屈服强度与晶粒尺寸d 成线性关系：σs=σi + Ky*d0.5（σi=85.2，Ky=6.49）[18].前文通过A、C 两种路径模拟了AZ31 镁合金经ECAP 变形后的材料内部的应变关系.经查阅数据4 道次时相关参数K0=4.6，K1=0.049，K2=10，σi=85.2，Ky=6.49 带入式（3）得：
(4) 因此可以得到四道次后晶粒尺寸与材料内部应变的关系.A 路径4 道次后累积的应变为4.66；C 路径4道次后累积的应变为4.29.而实验中4 道次后得到的晶粒尺寸是2.5 μm，将其带入式（4），得出ε=4.59，与模拟分析的基本一致.因此可以认为此公式对AZ31镁合金适用.
3 结论
（1） 不同的模具拐角、模具圆心角和摩擦因子等对挤压件的应力应变分布、载荷分布及其大小有不同的影响，研究不同工艺参数对挤压件的影响规律，对于获得性能优良、组织均匀的工件具有重要意义.
（2） 通过分析金属变形行为、应变场和挤压件不同挤压路径时应变均匀性分布，发现经过4 道次的挤压后，AZ31 镁合金不仅晶粒得到细化，材料硬度，强度和延伸率也得到了提高.对于多道次挤压来说，发现一条较好的、能够得到均匀的等效应变分布的路径是十分必要的.
（3） 镁合金AZ31 的晶粒细化后的尺寸和力学性能可以通过宏观变形的累积表征来预测.结合改进的模型和微观模拟部分后，可以得到材料外部微观变形、内部微观机制和力学性能的关系.
（4） 通过应变累积可以预测超细晶镁合金的平均晶粒大小和力学性能.如果上述方法能够被大量的实验证明，则可广泛地应用于预测经ECAP 组织结构细化后各种材料的平均晶粒尺寸和力学性能.
-
![]()
图 2 （CF）n的拉曼光谱激光波长633 nm；激光功率18 mW；狭缝宽度200 μm
Fig 2. Raman spectra of (CF)n Laser wave length 633 nm; laser power 18 mW; slit width 200 μm
![]()
图 3 （CF）n热分解的非等温TG曲线和对应的α-T关系曲线
Fig 3. Non-isothermal TG curves of（CF）n thermal decomposition and corresponding α-T curves
![]()
图 4 各转化率α下ln（βdα/dT）i-1/Ti的线性关系
Fig 4. Linear relation of ln(βdα/dT)i-1/Ti for each extent of conversion α
![]()
图 5 等转化率活化能Eα与转化率α的关系
Fig 5. Dependence of isoconversion activation energy (Eα) on the extent of conversion (α)
![]()
图 6 体系中可能发生的气态产物转化反应的ΔG-T关系
Fig 6. ΔG-T relationships of the possible gaseous product reactions in the system
![]()
图 8 ln[（βdα/dT）exp（E/RT）]-ln[αm/n（1-α）]线性关系
Fig 8. Linear relationship of ln[(βdα/dT)exp(E/RT)]-ln[αm/n(1-α)]
![]()
图 10 （CF）n热分解的非等温动力学模拟验证
Fig 10. Simulation and verification for the non-isothermal kinetics of (CF)n thermal decomposition
![]()
图 11 853 K温度下（CF）n分解的TG曲线及等温动力学预测结果
Fig 11. TG curve of (CF)n decomposition and the result of isothermal kinetic prediction at 853 K
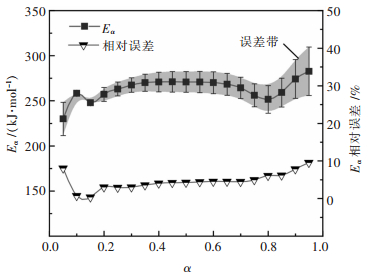
表 1 ln（βdα/dT）i-1/Ti线性关系的拟合结果及计算的Eα值
Table 1 Linear fitting results of ln(βdα/dT)i-1/Ti relationships and calculated Eα values
下载: 导出CSV
-
[1] NAKAJIMA T. Fluorine-carbon and fluoride-carbon materials: chemistry, physics and applications[M]. New York: Marcel Dekker, 1995: 1-31.
[2] SATO Y, ITOH K, HAGIWARA R, et al. Short-range structures of poly (dicarbon monofluoride) (C2F)n and poly (carbon monofluoride) (CF)n[J]. Carbon, 2004, 42(14): 2897-2903. doi: 10.1016/j.carbon.2004.06.042
[3] LAZAR P, OTYEPKOVÁ E, KARLICKY F, et al. The surface and structural properties of graphite fluoride[J]. Carbon, 2015, 94: 804-809. doi: 10.1016/j.carbon.2015.07.064
[4] THOMAS P, DELBÉ K, HIMMEL D, et al. Tribological properties of low-temperature graphite fluorides. Influence of the structure on the lubricating performances[J]. Journal of Physics & Chemistry of Solids, 2006, 67(5/6): 1095-1099. http://www.sciencedirect.com/science/article/pii/S002236970600045X
[5] MIAO X, YANG J, PAN W, et al. Graphite fluoride as a cathode material for primary magnesium batteries with high energy density[J]. Electrochimica Acta, 2016, 210: 704-711. http://www.sciencedirect.com/science/article/pii/S0013468616312877
[6] 房亚楠, 刘栓, 赵文杰, 等.石墨/氟碳涂层与氟化石墨/氟碳涂层腐蚀行为的研究[J].电镀与涂饰, 2016, 35(14): 747-754. http://www.cnki.com.cn/Article/CJFDTOTAL-DDTL201614011.htm [7] 康文泽, 李尚益.氟化石墨烯制备与研究进展[J].炭素, 2016(3): 12-16. http://d.old.wanfangdata.com.cn/Periodical/ts201603002 [8] 黄海平, 徐亮, 岳亚锋, 等.基于石墨烯量子点修饰电极的亚硝酸根电化学传感器[J].有色金属科学与工程, 2017, 8(2): 47-51. http://ysjskx.paperopen.com/oa/DArticle.aspx?type=view&id=2017020008 [9] CRASSOUS I, GROULT H, LANTELME F, et al. Study of the fluorination of carbon anode in molten KF-2HF by XPS and NMR investigations[J]. Journal of Fluorine Chemistry, 2009, 130(12): 1080-1085. doi: 10.1016/j.jfluchem.2009.07.022
[10] ZHU H M, SADOWAY D R. Anode reaction in aluminium electrolysis prior to and during anode effect[C]//ANJIER J L. Light Metals 2003. New York: John Wiley & Sons, Inc., 2003: 343-349.
[11] HAVERKAMP R G. An XPS study of the fluorination of carbon anodes in molten NaF-AlF3-CaF2[J]. Journal of Materials Science, 2012, 47(3): 1262-1267. doi: 10.1007/s10853-011-5772-5
[12] CHEN G, SHI Z N, WANG Z W, et al. Mechanism of graphite electrode fluorinated in 2.4 NaF/AlF3-Al2O3 melt at 1 373 K[J]. Journal of the Electrochemical Society, 2014, 161(14): C587-C593. doi: 10.1149/2.0781414jes
[13] WATANABE N, KOYAMA S, IMOTO H. Thermal decomposition of graphite fluoride. Ⅰ. Decomposition products of graphite fluoride, (CF)n in a vacuum[J]. Bulletin of the Chemical Society of Japan, 1980, 53(10): 2731-2734. doi: 10.1246/bcsj.53.2731
[14] BETTINGER H F, PENG H. Thermolysis of fluorinated single-walled carbon nanotubes: Identification of gaseous decomposition products by matrix isolation infrared spectroscopy[J]. Journal of Physical Chemistry B, 2005, 109(49): 23218-23224. doi: 10.1021/jp054370r
[15] CHEN G, SHI Z, YU J, et al. Kinetic analysis of the non-isothermal decomposition of carbon monofluoride[J]. Thermochimica Acta, 2014, 589(10): 63-69. http://www.sciencedirect.com/science/article/pii/S0040603114001993
[16] KAMARCHIK P, MARGRAVE J L. A study of thermal decomposition of the solid-layered fluorocarbon, poly (carbon monofluoride)[J]. Journal of Thermal Analysis, 1977, 11(2): 259-270. doi: 10.1007/BF01909964
[17] WATANABE N, KOYAMA S. Thermal decomposition of graphite fluoride. Ⅱ. Kinetics of thermal decomposition of (CF)n in a vacuum[J]. Bulletin of the Chemical Society of Japan, 1980, 53(11): 3093-3099. doi: 10.1246/bcsj.53.3093
[18] 杨序纲, 吴琪琳.拉曼光谱的分析与应用[M].北京:国防工业出版社, 2008: 158-163. [19] VYAZOVKIN S, BURNHAM A K, CRIADO J M, et al. ICTAC kinetics committee recommendations for performing kinetic computations on thermal analysis data[J]. Thermochimica Acta, 2011, 520(1): 1-19. http://www.sciencedirect.com/science/article/pii/S0040603111002152
[20] VYAZOVKIN S. Model-free kinetics: staying free of multiplying entities without necessity[J]. Journal of Thermal Analysis & Calorimetry, 2006, 83(1): 45-51. doi: 10.1007-s10973-005-7044-6/
[21] 吴易燃, 姜恒, 宫红, 等.氢氧化镧的热分解动力学[J].稀土, 2015, 36(4): 116-119. http://www.cnki.com.cn/Article/CJFDTOTAL-XTZZ201504022.htm [22] MÁLEK J. The kinetic-analysis of nonisothermal data[J]. Thermochimica Acta, 1992, 200(8): 257-269. http://www.sciencedirect.com/science/article/pii/004060319285118F
[23] KHAWAM A, FLANAGAN D R. Solid-state kinetic models: basics and mathematical fundamentals[J]. Journal of Physical Chemistry B, 2006, 110(35): 17315-17328. doi: 10.1021/jp062746a
[24] ŠESTÁK J, BERGGREN G. Study of the kinetics of the mechanism of solid-state reactions at increasing temperature[J]. Thermochimica Acta, 1971, 3(1): 1-12. doi: 10.1016/0040-6031(71)85051-7
[25] ŠIMON P. Fourty years of the Šesták-Berggren equation[J]. Thermochimica Acta, 2011, 520(1): 156-157.
[26] 胡荣祖.热分析动力学[M]. 2版.北京:科学出版社, 2008: 163-164. -
期刊类型引用(3)
1. 石勇，郑伯坤，段弘宇，黄腾龙，彭亮，赖伟，廖舟. 桨叶复合结构对充填料浆均质化的影响研究. 矿业研究与开发. 2024(06): 149-155 . 
百度学术
2. 高晨育，李强. 双层桨搅拌釜气液两相流的混合效果研究. 化工机械. 2024(04): 546-549+633 . 百度学术
3. 王忠锋，冯羽生，黄伟玲. 不同结构组态对导流筒搅拌槽混合效率影响分析. 有色金属科学与工程. 2024(06): 814-821+855 . 本站查看
其他类型引用(5)
计量
- 文章访问数: 92
- HTML全文浏览量: 20
- PDF下载量: 9
- 被引次数: 8
