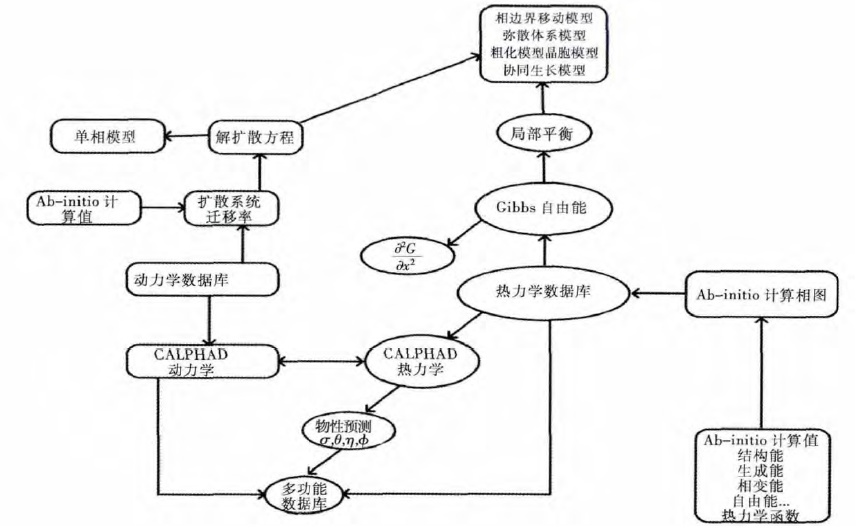
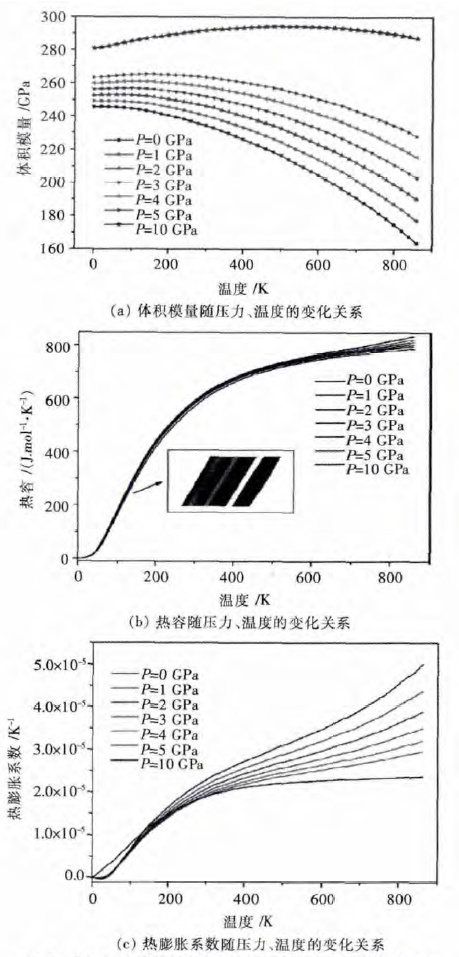
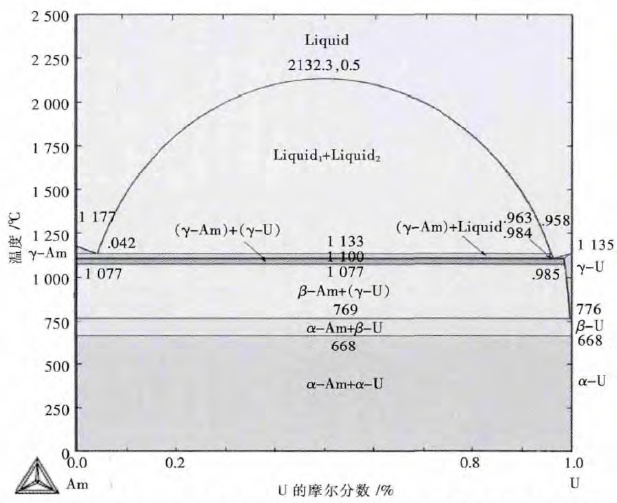
Application progress of first-principles calculations in CALPHAD technology
-
摘要: 相图计算 （Calculation of Phase Diagram, CALPHAD)是冶金 、化工 、材料设计等各个领域的重要研究工具，随着传统材料体系的研究深入和新材料体系的不断被发现，相关的相图和热力学实验数据愈显匮乏.基于电子层面的第一性原理计算（First-principles Calculations)，可以从体系基态性质出发，研究亚稳相、金属间化合物的结构、合金的稳定性，促进 CALPHAD 的发展.文中关注了近年来第一性原理计算在 CALPHAD 技术当中的应用研究进展，从计算化合物的能量性质，相图，动力学性质等方面进行系统阐述.Abstract: CALPHAD(Calculation of Phase Diagram) technology plays an important role in the fields of metal- lurgy, chemical engineering and material design. Phase diagram and thermodynamics data are really rare with the deepened study of traditional materials and discovery of new compounds. First -principles calculations based on electronic level boost the development of CALPHAD technology which can study the structure of metastable phase and intermediate compound and the stability of alloys from the perspective of system ground- state properties. The paper summarized the application progress of first-principles calculations in CALPHAD technology, and systematically expounded the calculations of energy properties of the compound, phase diagram and dynamical properties.
-
Keywords:
- CALPHAD /
- first-principles calculations /
- energy properties /
- phase diagram /
- dynamical properties
-
0 前言
铝土矿用碱溶后得出的矿浆由铝酸钠溶液和赤泥组成, 必须将二者分离, 以获得符合晶种分解要求的纯净溶液。分离后的赤泥要经过洗涤, 尽可能减少以附液形式损失于赤泥中的Na2O和Al2O3。因此, 赤泥沉降分离、洗涤是氧化铝生产中必需的工艺过程。沉降槽能否稳定运行直接关系到拜尔法的正常生产, 影响沉降槽稳定运行的因素有很多。笔者收集山西铝厂Ø42m沉降槽多年的部分生产实践数据, 论述影响Ø42m沉降槽稳定运行的几个关键因素, 对于拜尔法生产具有实际意义。
1 矿物特性及石灰用量对沉降性能的影响
(1) 生产实践证明, 铝土矿物组成和化学成分是影响赤泥浆液沉降、压缩性能的主要因素。铝土矿中, 常见黄铁矿、针铁矿、高岭石、金红石等矿物生成的赤泥, 这些矿物具有亲水性, 能降低赤泥沉降速度。其中, 高岭石在溶出过程中生成亲水性很强的含水铝硅酸钠沉淀, 不利于沉降。当原矿中SiO2含量高于10%时, 赤泥的沉降性能和压缩性能都很差。石英对赤泥沉降速度的影响比高岭石小。矿物中的有机物多, 赤泥浆液粘度越大, 赤泥沉降速度越慢, 而赤泥矿、菱铁矿、磁铁矿、锐钛矿等则有利于赤泥沉降。
(2) 石灰添加量对沉降性能的影响。适当添加石灰使溶出速度加快, 提高氧化铝溶出率, 还可降低赤泥中碱含量, 但添加过量反而造成Al2O3溶出率下降, 增加赤泥量。针铁矿型铝土矿加入CaO还会生成赤铁矿泥渣, 石灰还可使方钠石转变为钙霞石, 并减小了赤泥比表面, 石灰可以吸附一些有机物, 主要是草酸盐, 因而使赤泥沉降性能有较明显的改善。需要指出的是严禁使用没烧透的石灰石, 因为石灰石会严重影响赤泥沉降性能。生产中石灰加入量要根据反应条件、矿石中针铁矿含量、矿物中TiO2含量、石灰活性、碱耗等因素考虑确定。
2 溶出效果对沉降性能的影响
溶出效果的好坏是影响沉降槽稳定运行的关键因素, 影响溶出效果的因素很多, 如合格矿浆、溶出温度、反应时间、搅拌强度、满罐率等都能影响溶出效果。效果好时, 产生赤泥量少, 赤泥沉降压缩性能好, 就为沉降槽稳定运行创造了条件, 否则溶出效果不好时, 铝土矿没有充分反应, 赤泥沉降性能差, 严重时沉降速度几乎没有, 导致沉降槽跑浑。山西铝厂1997年6月中旬至6月底, 就是溶出效果差、溶出率低、赤泥量大、赤泥沉降性能差, 导致沉降槽半个月运行不稳定。1997年至1998年4月溶出矿浆月平均Na2OT浓度272.2g/L, Al2O3浓度267.9g/L, Na2Ok浓度244.4g/L, 固体含量113g/L, αk1.50, 上述结果表明:溶出效果好时, Al2O3浓度均在250 g/L以上, 固体含量较低(为110g/L以下), αk较低(为1.47~1.52)。
总之, 配料后溶出效果好坏是影响沉降槽能否稳定运行的首要因素。
3 稀释浓度对沉降性能的影响
山西铝厂溶出后矿浆Al2O3浓度一般都在250 g/L以上, 固体含量在110g/L左右, 这种浓赤泥浆液粘度大, 直接分离非常困难, 实际上是不能进行的。稀释的结果, 使溶液浓度降到中等浓度, 浓度降低, 比重下降, 且赤泥溶剂化程度降低, 促进了粒子聚结。因而, 赤泥沉降速度增加, 分离洗涤效率提高, 图 1为30℃下溶液浓度与粘度的关系的变化曲线〔1〕。由图 1可见, 铝酸钠溶液粘度与浓度关系很大。
为了减少蒸发负担, 精液Al2O3浓度应控制在170g/L左右, 稀释Al2O3浓度要控制在175g/L左右。生产实践证明, 浓度越高, 沉降速度越低, 当Al2O3浓度高于200g/L时, 沉降速度在0.3~0.4m/h, 对沉降槽操作极为不利。稀释浓度控制在160~180g/L为宜。
4 除砂对沉降槽的影响
赤泥中大于150μm的大颗粒往往称之为砂。由于赤泥在沉降槽中的沉降过程属于重力沉降, 沉降的速度与赤泥粒径的平方成正比, 沉降槽运行过程, 大粒砂子不仅沉降速度快而在槽底沉积, 增大沉降槽负荷, 影响沉降槽稳定运行, 而且增大沉降槽进料固体含量和总泥量, 影响沉降槽的生产能力。此外, 大量粗砂很容易在赤泥过滤机半月槽中形成沉淀, 影响赤泥过滤机的正常运行, 生产中发现, 大量的砂子对底流泵、饲料泵、滤饼泵、赤泥泵的过流件磨损加快, 并影响这些设备的检修周期。所以, 除去进入沉降槽的粗砂是改善沉降槽的运行条件和提高生产能力的有效措施。
生产上采用水力旋流器和分级机把粗砂除去, 效果明显。
5 温度对沉降性能的影响
铝酸钠溶液的粘度随温度升高而减小。铝酸钠溶液粘度的对数与绝对温度的倒数呈直线关系, 即lgη=f(1/T)为直线关系〔1〕, 见图 2。
![]()
图 2 NaOH浓度为125g/L的铝酸钠溶液lgη=f(1/T)关系1.溶液Al2O3浓度100g/L; 2.溶液Al2O3浓度75g/L; 3.溶液Al2O3浓度50g/L; 4.溶液Al2O3浓度25g/L赤泥沉降过程是液固分离过程, 温度越高, 粘度越小, 赤泥沉降速度越快。另外, 温度高, 溶液越稳定, 氧化铝水解损失少, 所以保证沉降槽温度至关重要。生产实践上, 要求分离温度不低于95 ℃, 洗涤温度不低于90 ℃。
溶出开车运转期间, 分离温度都能保证在100 ℃左右, 可满足工艺要求。但溶出停车期间, 分离槽温度会很快降低。生产中发现:分离槽温度一旦低于90 ℃, 粗液送往叶滤, 叶滤机布会很快结硬, 影响生产。目前, 采取措施是溶出停车, 尽快把粗液送走, 把分离槽液位降到最低, 溶出出料后, 把料存在分离槽, 利用溶出来料把分离温度提到95℃以上, 再给叶滤送料, 这种方法切实可行。
6 絮凝剂质量和添加量对沉降性能的影响
赤泥沉降过程中添加絮凝剂是目前工业上普遍采用的且行之有效的加速赤泥沉降的办法。在絮凝剂作用下, 处于分散状态的小赤泥颗粒互相联合成团, 粒度增大, 使沉降速度大大增加。对于高分子絮凝剂作用机理, 一般认为絮凝剂的作用可划分为吸附和絮凝两个阶段。絮凝剂吸附于固体粒子表面是絮凝剂作用的必要条件和关键, 但吸附并不一定都能导致有效的絮凝作用, 只是在固体粒子表面吸附了某种适宜数量的絮凝剂时, 才能进行有效的絮凝。因此, 絮凝剂质量和添加量一定要满足工艺要求。
山西铝厂Ø42m沉降槽, 在1997年曾用过常见的4种絮凝剂:天然絮凝剂(麦麸)、英国絮凝剂ALCLAR600、河南韦辉絮凝剂PAS-1、山西铝厂的PPR-Na1000(胶体)。
下面就以上述4种絮凝剂在42m沉降槽使用情况作比较。
(1) 天然絮凝剂即麦麸, 由于它对细小颗粒沉降起较大作用, 现只用在分离槽上, 用量没有固定数量, 视分离槽状况适时调整, 一般配制浓度在0.01%~0.02%, 由于可以边配制边加入, 在分离槽出现跑浑时, 可以加大量, 短时间内即可见效(针对因絮凝剂加入量小而引起的跑浑)。
(2) 英国的ALCLAR600, 其分离、洗涤均可用, 配制浓度一般在0.025%, 最大优点是澄清度好、溶解时间短、搅拌1h即可, 沉降速度一般为0.5~1.0 m/h, 赤泥压缩性能也较好, 半小时后液固比可达到2左右, 配制时需分散加入, 防止出现“鱼眼”。
(3) PAS-1, 分离洗涤均可用, 配制浓度和英国ALCLAR600一样, 为0.025 %, 最大优点是沉降速度快, 可以达到1~2m/h, 是ALCLAR600和PPR-Na1000的2倍以上, 10min液固比即可达到2以上, 溶解也较快, 搅拌1~2h即可, 缺点是10min内清液层不清, 20min方可澄清, 配制时需要一点一点地分散加入, 防止出现“鱼眼”。
(4) PPR-Na1000分离洗涤均可使用, 配制浓度在0.016%~0.02 %, 沉降速度慢, 一般在0.5m/h左右, 赤泥压缩性能1h后液固比可达到2左右, 溶解时间长, 需搅拌4~5h, 配制时间长, 优点是澄清度好, 配制时可以迅速整袋倒入, 而不会生成“鱼眼”。
对絮凝剂加入量原则上在保证赤泥沉降速度和澄清度前提下要尽量少加。若加量过大会增大溶液中有机含量, 溶液粘度增大, 反而影响赤泥沉降和压缩性能, 甚至还会形成稳定性高、密度小的絮凝团, 不但自身不易沉降, 而且增大赤泥粒子沉降阻力, 沉降速度减小, 再则在赤泥浆中存在时间长, 还会引起赤泥变性。另外, 在分离槽中加入量过大, 絮凝剂随粗液带入叶滤机, 在叶滤机布上形成一层粘膜, 严重影响叶滤机处理量, 同时加入量大, 还会影响精种分解。生产上采用有效对策是在粗液中加入石灰乳, 效果明显。
生产上, 絮凝剂加入量不够是引起沉降槽跑浑的主要原因, 也是最常见的事故, 这种事故特点是发生得快, 处理得也快。目前, 随着溶出进料量不断增大, 生产上为解决絮凝剂加入量不够问题, 往往增大了絮凝剂配制浓度。生产需要注意的另一方面是絮凝剂配制, 必须注意两点:一是用水一定要清洁, 不要带料; 另一方面, 边配边用。
总之, 絮凝剂质量和添加量, 是影响沉降槽稳定运行的一个重要的因素。
7 工艺管理
对沉降工艺来说关键在于操作, 要根据各项指标进行调整, 并注意以下几点。
(1) 溶出进料量时要维持各分离槽进料相等均衡。由于分离槽进料量是靠分料箱上的塞子开动调整的, 稀释后泵开动不一样, 会引起分离进料量变化, 通过塞子力争进料平衡。生产中发现, 出现进料不均衡, 进料多的容易跑浑, 这是因为进料多的超过了分离槽产能。
(2) 每2h测一次沉降速度, 如果溶出按900m3/h进料时, 两个分离槽每个要产粗液600m3/h左右, 分离槽面积1 380m2, 据此来料沉降速度至少达到0.44m/h方能保证粗液合格, 发现沉降速度不好, 要从来料、絮凝剂等方面寻找原因, 及时调整。最常见的办法是加大麦麸, 调大合成絮凝剂。
(3) 泥层调整, 每2h测量一次泥层, 正常情况下, 分离泥层控制在2m以下, 一洗2.5m(溢流有可能浑浊), 分离可适当拉底流, 但分离泥层不要超过2.5m, 超过2.5m分离有可能造成溢流浑浊。
(4) 分离底流固体含量控制在300~400g/L之间, 为了提高洗涤效率, 不要低于300g/L, 也不要超过450g/L, 拉分离底流要兼顾一洗Na2OT浓度, 以60~80g/L为宜, 低于60g/L, 容易造成分离积泥; 超过80g/L, 会造成一洗浓度高, 赤泥沉降性能下降。
(5) 要力求提高末次底流固体含量, 因为只有提高末次固体含量, 赤泥过滤机产能提高, 沉降槽的泥才能及时排走。
(6) 分离槽尽可能低料位控制, 一方面可以有较大缓冲能力, 另一方面出现跑浑容易处理。洗涤槽控制在5.0m左右, 一方面保证溶出洗液易供应, 另一方面, 洗涤槽溢流箱内容易积泥, 高料位可以保证周边出料。
(7) 过滤两段滤饼槽、饲料槽不要冒槽, 过滤返回沉降赤泥不易沉降, 尤其是碱赤泥影响更大。
-
![]()
图 1 第一性原理计算在CALPHAD 技术中的应用流程
Fig 1. Application of first principle calculation in CALPHAD
![]()
图 3 不同压强下AlSc2的热力学性质随温度的变化关系
Fig 3. Dependence of thermodynamic properties of AlSc2 on temperature and pressure
![]()
图 4 立方结构型W 在零压下的热力学性质随温度的变化
Fig 4. Dependence of thermodynamic properties of cubic structure W on temperature under zero pressure
![]()
图 5 Ti-Si-N 在不同压力及温度下的热力学性质比较
Fig 5. Dependence of thermodynamic properties of Ti-Si-N system on pressure and temperature
![]()
图 6 采用Ab initio 计算与CALPHAD 结合计算得到的Am-U 体系相图
Fig 6. Phase diagram of Am-U system calculated by combination of firstprinciples calculations and CALPHAD
![]()
图 7 ɑ-Fe 自扩散系数的第一性原理计算与实验结果比较
Fig 7. Comparison between first-principle calculation for self diffusion coefficients of ɑ-Fe and experimental result
-
[1] 乔芝郁, 郝士明. 相图计算研究的进展[J]. 材料与冶金学报, 2005, 4(2): 83-90. http://www.cnki.com.cn/Article/CJFDTOTAL-HUJI200502005.htm [2] 郭景坤. 陶瓷晶界应力设计[J]. 无机材料学报, 1995, 10(1): 27-31. http://www.cnki.com.cn/Article/CJFDTOTAL-WGCL501.005.htm [3] Saunders N, Miodownik A P. CALPHAD (calculation of phase diagrams): A comprehensive guide[M]. Elsevier: Elsevier, 1998.
[4] Ye X Y, Zhang J, Wang Y, et al. Optimization and calculation of the LaBr3-MBr (M= Na, K, Rb, Cs) phase diagrams[J]. Calphad, 2004, 28(2): 147-151. doi: 10.1016/j.calphad.2004.07.006
[5] Ye X Y, Zhang J, Sun Y M, et al. Thermodynamic optimization of TmCl3-ACl (A= Na, K, Rb, Cs) phase diagrams [J]. Rare Earths, 2005, 23(1): 88-92. http://cn.bing.com/academic/profile?id=fea13758912505fbc7d48f267b473311&encoded=0&v=paper_preview&mkt=zh-cn
[6] Ye X Y, Sun Y M, Zhang J, et al. Thermodynamic optimization of DyCl3-NaCl system[J]. Journal of Shanghai University (English Edition), 2005, 9(3): 279-282. doi: 10.1007/s11741-005-0093-z
[7] Ye X Y, Zhuang W D, Deng C Y, et al. Thermodynamic investigation on the Al2O3-BaO binary system[J]. Calphad,2006, 30(3): 349-353. doi: 10.1016/j.calphad.2005.12.004
[8] Ye X Y, Zhuang W D, Wang J F, et al. Thermodynamic description of SrO-Al2O3 system and comparison with similar systems[J]. Journal of Phase Equilibria and Diffusion, 2007, 28(4): 362-368. doi: 10.1007/s11669-007-9086-x
[9] Tao X, Ouyang Y, Liu H, et al. Abinitio calculation of the total energy and elastic properties of Laves phase C15 Al2RE (RE= Sc, Y, La, Ce-Lu)[J]. Computational Materials Science, 2008, 44(2): 392-399. doi: 10.1016/j.commatsci.2008.03.036
[10] Kaufman L, Bernstain H. Computer calculation of phse diagram[M]. New York: Academic Press,1970.
[11] Hillert M. Phase equilibria, phase diagram and phase transformations[M]. Cambridge University: Cambridge,1998.
[12] Segall M D, Lindan P J D, Probert M J, et al. First-principles simulation: Ideas, illustrations and the CASTEP code[J]. Journal of Physics: Condensed Matter, 2002, 14(11): 2717. doi: 10.1088/0953-8984/14/11/301
[13] 谢希德, 陆栋. 固体能带理论[M]. 上海: 复旦大学出版社, 1998. [14] Fermi E. Eine statistische methode zur bestimmung einiger eigenschaften des atoms und ihre anwendung auf die theorie des periodischen systems der elemente[J]. Zeitschrift für Physik,1928, 48(1/2): 73-79. http://cn.bing.com/academic/profile?id=824db6f9ae6f53dbe040a36ee89a28c6&encoded=0&v=paper_preview&mkt=zh-cn
[15] Kohn W. Nobel lecture: Electronic structure of matter-wave functions and density functionals[J]. Reviews of Modern Physics,1999, 71(5): 1253-1266. doi: 10.1103/RevModPhys.71.1253
[16] Kohn W, Sham L J. Quantum density oscillations in an inhomogeneous electron gas[J]. Physical Review,1965, 137(6A): A1697-A1705. doi: 10.1103/PhysRev.137.A1697
[17] Thomas L H. The calculation of atomic fields[C]// Cambridge: Cambridge University Press, 1927: 542-548.
[18] Hohenberg P, Kohn W. Inhomogeneous electron gas[J]. Physical Review, 1964, 136(3B): B864. doi: 10.1103/PhysRev.136.B864
[19] Kohn W, Sham L J. Self-consistent equations including exchange and correlation effects[J]. Physical Review, 1965, 140(4A): A1133-A1138. doi: 10.1103/PhysRev.140.A1133
[20] Slater J C. A simplification of the Hartree-Fock method[J]. Physical Review, 1951, 81(3): 385-391. doi: 10.1103/PhysRev.81.385
[21] Ceperley D M, Alder B J. Ground state of the electron gas by a stochastic method[J]. Physical Review Letters,1980, 45(7): 566-573. doi: 10.1103/PhysRevLett.45.566
[22] Perdew J P, Zunger A. Self-interaction correction to density-functional approximations for many-electron systems[J]. Physical Review B, 1981, 23(10): 5048-5079. doi: 10.1103/PhysRevB.23.5048
[23] Perdew J P, Burke K, Ernzerhof M. Generalized gradient approximation made simple[J]. Physical review letters, 1996, 77(18): 3865-3868. doi: 10.1103/PhysRevLett.77.3865
[24] Perdew J P, Kurth S, Zupan A, et al. Accurate density functional with correct formal properties: A step beyond the generalized gradient approximation[J]. Physical review letters, 1999, 82(12): 2544-2549. doi: 10.1103/PhysRevLett.82.2544
[25] Lee C, Yang W, Parr R G. Development of the colle-salvetti correlation-energy formula into a functional of the electron density[J]. Physical review B, 1988, 37(2): 785-796. doi: 10.1103/PhysRevB.37.785
[26] X G. First-principles responses of solids to atomic displacements and homogeneous electric fields: Implementation of a conjugate-gradient algorithm[J]. Physical Review B, 1997, 55(16): 10337-10354. doi: 10.1103/PhysRevB.55.10337
[27] X G. A brief introduction to the ABINIT software package[J]. Zeitschrift für Kristallographie, 2005, 220(5/6): 558-562. http://cn.bing.com/academic/profile?id=0cf434abb21627c86a9911804f80c73e&encoded=0&v=paper_preview&mkt=zh-cn
[28] Gonze X, Amadon B, Anglade P M, et al. ABINIT: First-principles approach to material and nanosystem properties[J]. Computer Physics Communications,2009, 180(12): 2582-2615. doi: 10.1016/j.cpc.2009.07.007
[29] Martin R M. Electronic structure: basic theory and practical methods[M]. Cambridge : Cambridge University Press, 2004.
[30] Kresse G, Furthmüller J. Software VASP, vienna (1999)[J]. Physics Review B, 1996, 54(11): 169.
[31] Soler J M, Artacho E, Gale J D, et al. The SIESTA method for ab initio order -N materials simulation[J] . Journal of Physics: Condensed Matter,2002, 14(11): 2745-2779. doi: 10.1088/0953-8984/14/11/302
[32] Schwarz K, Blaha P, Madsen G K H. Electronic structure calculations of solids using the WIEN2k package for material sciences[J]. Computer Physics Communications, 2002, 147(1): 71-76. http://cn.bing.com/academic/profile?id=f4d4c71637dba08b2633ef1cfdcbb86b&encoded=0&v=paper_preview&mkt=zh-cn
[33] Van de Walle A, Asta M, Ceder G. The alloy theoretic automated toolkit: A user guide[J]. Calphad, 2002, 26(4): 539-553. doi: 10.1016/S0364-5916(02)80006-2
[34] Kresse G, Joubert D. From ultrasoft pseudopotentials to the projector augmented-wave method[J]. Physical Review B, 1999, 59(3): 1758-1775. doi: 10.1103/PhysRevB.59.1758
[35] Vanderbilt D. Soft self-consistent pseudopotentials in a generalized eigenvalue formalism[J]. Physical Review B, 1990, 41(11): 7892-7895. doi: 10.1103/PhysRevB.41.7892
[36] Wood D M, Zunger A. A new method for diagonalising large matrices[J]. Journal of Physics A: Mathematical and General,1985, 18(9): 1343-1359. doi: 10.1088/0305-4470/18/9/018
[37] Davidson E R. The iterative calculation of a few of the lowest eigenvalues and corresponding eigenvectors of large real-symmetric matrices[J]. Journal of Computational Physics, 1975, 17(1): 87-94. doi: 10.1016/0021-9991(75)90065-0
[38] Pulay P. Convergence acceleration of iterative sequences. The case of SCF iteration[J]. Chemical Physics Letters, 1980, 2: 73. http://cn.bing.com/academic/profile?id=f57404acf93c3922cf17f379d899e537&encoded=0&v=paper_preview&mkt=zh-cn
[39] Pulay P. Improved SCF convergence acceleration[J]. Journal of Computational Chemistry,1982, 3(4): 556-560. doi: 10.1002/(ISSN)1096-987X
[40] D J D. Modified Broyden's method for accelerating convergence in self-consistent calculations[J]. Physical Review B, 1988, 38(18): 12807-12812. doi: 10.1103/PhysRevB.38.12807
[41] Kaufman L, Turchi P E A, Huang W, et al. Thermodynamics of the Cr-T-W system by combining the Ab initio and CALPHAD method[J]. Calphad, 2001, 25(3): 419-433. doi: 10.1016/S0364-5916(01)00061-X
[42] Colinet C. Phase diagram calculations: contribution of ab initio and cluster variation methods[M].USA: 2002.
[43] 陈星秋, 严新林, 丁学勇, 等. 化合物生成焓:一百年和密度泛函基量子机制的原子模型新时代[J]. 中国稀土学报, 2004, 22: 1-7. [44] 赵燕燕. Ag-Cu-Ni-Ce合金系二元稀土化合物的第一性原理计算研究[D]. 重庆: 重庆理工大学, 2014. [45] 赵沙斐, 潘荣凯, 周思晨, 等. Al4Ce和Al2CeZn2相弹性性能的第一性原理研究[J]. 郑州大学学报(工学版), 2014(2): 104-107. http://www.cnki.com.cn/Article/CJFDTOTAL-ZZGY201402024.htm [46] 曾梦雪. Mg2Y(Ca)和tl26型Mg12RE合金的弹性性能与电子结构研究[D]. 南宁: 广西大学, 2012. [47] 苏昕. 稀土镁合金的第一性原理计算及组织性能研究[D]. 上海: 上海交通大学, 2013. [48] Chen G, Zhang P. First-principles study of electronic structures, elastic properties and thermodynamics of the binary intermetallics in Mg-Zn-Re-Zr alloy[J]. Defence Technology, 2013, 9(3): 131-139. doi: 10.1016/j.dt.2013.09.011
[49] 王文静, 刘子利, 刘希琴, 等. Mg-Er金属间化合物稳定性与电子结构的第一性原理研究[J]. 中国有色金属学报, 2014, 24(2): 7-9. http://www.cnki.com.cn/Article/CJFDTOTAL-ZYXZ201402007.htm [50] Yang Q, Liu X, BU F, et al. First-principles phase stability and elastic properties of Al-La binary system intermetallic compounds[J]. Intermetallics, 2015, 60: 92-97. doi: 10.1016/j.intermet.2015.02.007
[51] 邹祥宇, 廖先杰, 卫学玲, 等. Al3Sc的FFC法制备和第一性原理计算研究[J]. 材料与冶金学报, 2014, 13(4): 284-287. http://www.cnki.com.cn/Article/CJFDTOTAL-HUJI201404012.htm [52] Pang M, Zhan Y, Wang H, et al. Ab initio investigation of structural, electronic, mechanical, and thermodynamic properties of AlSc2 intermetallic compound under pressure[J]. Journal of Applied Physics, 2011, 110(3): 33533-33534. doi: 10.1063/1.3622340
[53] Zhang Y, Kresse G, Wolverton C. Nonlocal first-principles calculations in Cu-Au and other intermetallic alloys[J]. Physical Review Letters, 2014, 112(7): 75502-75503. doi: 10.1103/PhysRevLett.112.075502
[54] 温玉锋, 孙坚, 黄健. 基于特殊准随机结构模型的FCC Fe-Cu无序固溶体合金的弹性稳定性[J]. 中国有色金属学报, 2012, 22(9): 2522-2528. http://www.cnki.com.cn/Article/CJFDTOTAL-ZYXZ201209016.htm [55] Cheng H C, Yu C F, Chen W H. Physical, mechanical, thermodynamic and electronic characterization of Cu11In9 crystal using first-principles density functional theory calculation[J]. Computational Materials Science, 2014, 81: 146-157. doi: 10.1016/j.commatsci.2013.07.039
[56] Du J, Wen B, Melnik R, et al. Phase stability, elastic and electronic properties of Cu-Zr binary system intermetallic compounds: A first-principles study[J]. Journal of Alloys and Compounds, 2014, 588: 96-102. doi: 10.1016/j.jallcom.2013.11.018
[57] Zhang Y, Ozolins V, Morelli D, et al. Prediction of new stable compounds and promising thermoelectrics in the Cu-Sb-Se system[J]. Chemistry of Materials, 2014, 26(11): 3427-3435. doi: 10.1021/cm5006828
[58] 庞梦德. Be-Sr, Be-Mg二元系和C-Co-W-Nb四元系的热力学研究[D]. 长沙: 中南大学, 2014. [59] 黄多辉, 李强, 曹启龙, 等. W热力学性质和弹性性质的第一性原理研究[J]. 稀有金属材料与工程, 2013, 42(9): 1849-1853. http://www.cnki.com.cn/Article/CJFDTOTAL-COSE201309018.htm [60] 梁超平. 若干过渡族金属及合金系统关键性能的第一性原理研究[D]. 长沙: 中南大学, 2013. [61] 翟东, 韦昭, 冯志芳, 等. 铜钨合金高温高压性质的第一性原理研究[J]. 物理学报, 2014, 63(20): 206501. http://www.cnki.com.cn/Article/CJFDTOTAL-WLXB201420037.htm [62] Tan X, Wang Y, Jia Y, et al. Ab-initio study of the structure and thermodynamic properties of Ti-Si-N at external pressure[J]. Computational Materials Science, 2015, 96: 33-38. doi: 10.1016/j.commatsci.2014.08.041
[63] Perron A, Turchi P E A, Landa A, et al. The Pu-U-Am system: An ab initio informed CALPHAD thermodynamic study[J]. Journal of Nuclear Materials, 2015, 458: 425-441. doi: 10.1016/j.jnucmat.2014.12.101
[64] Bittner R W, Colinet C, Tedenac J C, et al. Revision of the Ge-Ti phase diagram and structural stability of the new phase Ge4Ti5[J]. Journal of Alloys and Compounds, 2013, 577: 211-216. doi: 10.1016/j.jallcom.2013.04.114
[65] Mohri T, Chen Y. First-principles investigation of L10-disorder phase equilibria of Fe-Ni,-Pd, and -Pt binary alloy systems[J]. Journal of Alloys and Compounds, 2004, 383(1): 23-31. http://cn.bing.com/academic/profile?id=131ea6ea9bf75602f417b949ad4e58a6&encoded=0&v=paper_preview&mkt=zh-cn
[66] 罗伟. Ga-Zr和Co-Cr-Nb体系的相图测定和热力学计算[D]. 长沙: 中南大学, 2014. [67] Xiong W, Xie W, Morgan D. Thermodynamic evaluation of the Np-Zr system using CALPHAD and ab initio methods[J]. Journal of Nuclear Materials, 2014, 452(1): 569-577. http://cn.bing.com/academic/profile?id=19a4867d1704c154a980e41423d786dc&encoded=0&v=paper_preview&mkt=zh-cn
[68] Rajkumar V B, Kumar K C H. Thermodynamic modeling of the Fe-Mo system coupled with experiments and ab initio calculations[J]. Journal of Alloys and Compounds, 2014, 611: 303-312. doi: 10.1016/j.jallcom.2014.05.030
[69] Štrof ,J, Pavlů J, Wdowik U D, et al. Laves phases in the V-Zr system below room temperature: Stability analysis using ab initio results and phase diagram[J]. Calphad,2014, 44: 62-69.
[70] Berche A, Tédenac J C, Jund P. Ab-initio calculations and CALPHAD description of Cr-Ge-Mn and Cr-Ge-Si[J]. Calphad, 2015, 49: 50-57. doi: 10.1016/j.calphad.2015.02.004
[71] Saunders N, Miodownik A P. Calculation of phase diagrams (CALPHAD): a comprehensive guide[M]. Elsevier: Elsevier, 1998.
[72] Mantina M, Wang Y, Arroyave R, et al. First-principles calculation of self-diffusion coefficients[J]. Physical Review Letters, 2008, 100(21): 215901-215904. doi: 10.1103/PhysRevLett.100.215901
[73] Sandberg N, Chang Z, Olsson P, et al. Modeling of the magnetic free energy of self-diusion in bcc Fe[J]. Physical Review B, 2015,75(2): 1-8. http://cn.bing.com/academic/profile?id=3365a792de3d2260528d5dfd34febd49&encoded=0&v=paper_preview&mkt=zh-cn
[74] Van der Ven A, Ceder G. First principles calculation of the interdiffusion coefficient in binary alloys[J]. Physical Review Letters, 2005, 94(4): 45901. doi: 10.1103/PhysRevLett.94.045901
[75] Ganeshan S, Hector L G, Liu Z K. First-principles calculations of impurity diffusion coefficients in dilute Mg alloys using the 8-frequency model[J]. Acta Materialia, 2011, 59(8): 3214-3228. doi: 10.1016/j.actamat.2011.01.062
下载:
计量
- 文章访问数: 73
- HTML全文浏览量: 449
- PDF下载量: 46
