Numerical simulation of charged particle and electric field in electrical area of electrostatic filter bag
-
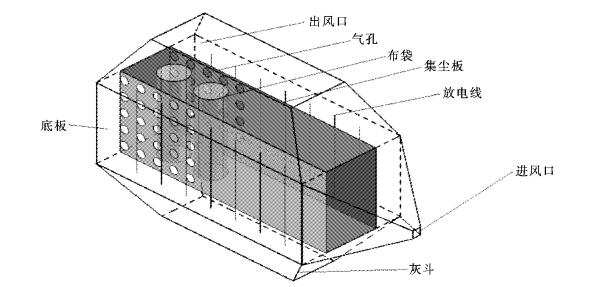
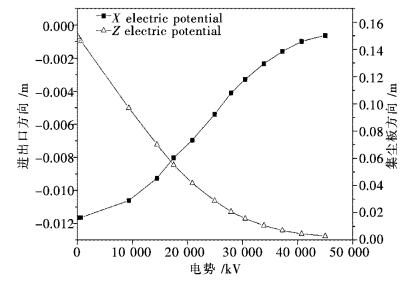
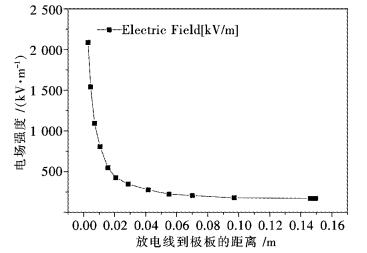
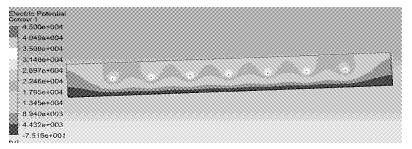
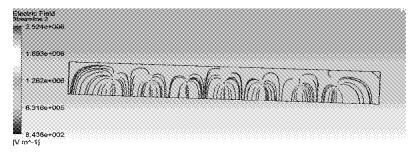
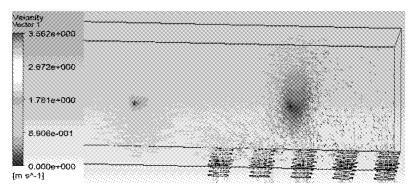
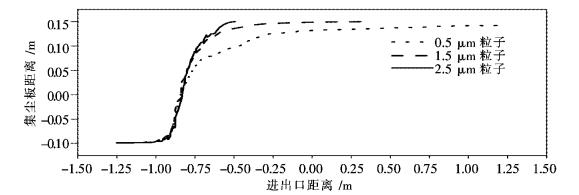
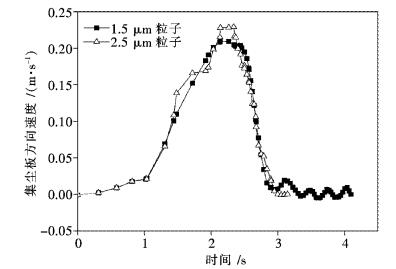
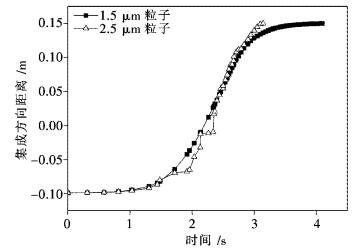
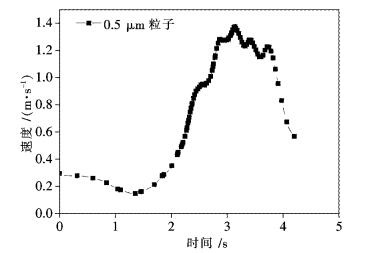
摘要: 运用FLUENT对电袋除尘器电场区域内流场、电晕电场、荷电粒子运动轨迹进行数值模拟, 确定静电区捕集粉尘的最小粒径, 优化静电区集尘板的最佳开孔范围.首先数值模拟了电袋除尘器静电区的流场分布和电场分布, 在此基础上, 分别数值模拟了粒径为0.5、1.5和2.5 μm的粒子在外加电压为45 kV的电场中的运动轨迹和速度分布, 并进行了数值分析.模拟结果表明:在该除尘器结构及模拟条件下, 除尘器静电区通道内最小捕集粒径为1.5 μm; 在静电区通道内集尘板X方向的最佳开孔范围是0.324~1.25 m.研究结果为电袋除尘器静电区内结构的设计和优化提供理论参考.Abstract: Numerical simulation are performed on the the flow field, electric field and charged particle trajectory in electrical area of EBP by using FLUENT software to work out the minimum removal diameter of dust in electric field and optimize the opening range of dust collector. Electric distribution and flow field distribution are numerically simulated. The particle movement and velocity distribution in the voltage of 45 kV in electric field, whose diameter includes 0.5, 1.5 and 2.5 μm, are numerically simulated and analyzed. The results show that the minimum removal diameter is 1.5 μm and the optimal opening ranges from 0.324 m to 1.25 m along the X direction in electrical passageway. The results can be used as theoretical reference for both electrostatic fabric filter design and structure optimization.
-
配置好的环烷酸，皂化后并不是简单的真溶液，而是水分散在油相中的微乳状液体系，萃取稀土时，上述液体系会使微乳状液破乳。通过串级萃取理论计算得出的有机相理论流量不夹带水份，实际生产中皂化好的环烷酸往往又夹带水份，因此，为达到正常的萃取分离效果，保证产品质量，需要对有坑相理论流量进行校正，即先估算一个百分数，但不可避免地使理论值与实际值有时出现偏差。
一 公式推导
今设：
Vs实 进槽有机相实际流量 1/min
Vs理 计算的有机相理论流量
ε 有机相皂化度
C 皂化有机相氨水浓度(N)
V1 未皂化的有机相体积(1)
V2 将V1皂化为皂化度ε的氨水体积
则：
(1) 
(2) 将式(2)代入式(1)得
(3) 令
(4) 
(5) 二 讨 论
1.系数k与有机相皂化度成正比，皂化度越大K值越大，反之亦然。K和皂化有机相的氨水浓度成反比。
2.公式(4)和(5)，适周于氨水皂化有机相时，水均匀分布于有机相中的任何体系。它的导出，为确定▽s实提供理论依据，为准确地计算整个槽体平衡提供可靠依据。
-
![]()
图 7 静电区无电场下气流分布
Fig 7. Airflow distribution of electrostatic area without electric field
![]()
图 9 不同粒径的粒子在电场中的运动轨迹
Fig 9. Movement locus of particles with different size in electric field
![]()
图 10 粒子在集尘板方向的速度变化
Fig 10. Change of particles' velocity in direction of integrated board
![]()
图 11 粒子在集尘板方向的运动距离变化
Fig 11. Change of particles' moving in direction of integrated board
-
[1] 易红宏, 郝吉明, 段雷, 等.电厂除尘设施对PM-(10)排放特征影响研究[J].环境科学, 2006(10): 1921-1927. http://www.cnki.com.cn/Article/CJFDTOTAL-HJKZ200610000.htm [2] Barranco R, Gong M, Thompson A, et al. The impact of fly ash resistivity and carbon content on electrostatic precipitator performance[J]. Fuel, 2007, 86(16): 2521-2527. doi: 10.1016/j.fuel.2007.02.022
[3] 岳勇, 陈雷, 姚强, 等.燃煤锅炉颗粒物粒径分布和痕量元素富集特性实验研究[J].中国电机工程学报, 2005(18): 74-79. http://www.cnki.com.cn/Article/CJFDTOTAL-ZGDC200518014.htm [4] Tanthapanichakoon W, Furuuchi M, Nitta K, et al. Degradation of semi-crystalline PPS bag-filter materials by NO and O2 at high temperature[J]. Polymer Degradation and Stability, 2006, 91(8): 1637-1644. doi: 10.1016/j.polymdegradstab.2005.12.008
[5] 龙正伟, 宋蔷, 李水清, 等.复合式电袋除尘器的伏安特性[J].中国电机工程学报, 2010 (14): 13-20. http://www.cnki.com.cn/Article/CJFDTOTAL-ZGDC201014004.htm [6] 李庆, 肖振雷, 李海风, 等.微细粉尘在静电除尘器中的运动状态分析[J].环境工程, 2011(2): 73-77. http://www.cnki.com.cn/Article/CJFDTOTAL-HJGC201102025.htm [7] 涂扬赓, 宋蔷, 涂功铭, 等.孔板对复合电袋除尘器静电区除尘性能影响的实验研究[J].中国电机工程学报, 2013, 33(17): 51-56. http://www.cnki.com.cn/Article/CJFDTOTAL-ZGDC201317009.htm [8] Kallio G A, Stock D E. Interaction of electrostatic and fluiddynamic fields in wire-plate electrostatic precipitators[J].Journal of Fluid Mechanics, l992, 240: 133-166. doi: 10.1017/S0022112092000053
[9] Liang W J, Lin T H. The characteristics of ionic wind andits effect on electrostatic precipitators[J]. Aerosol Science and Technology, 1994, 20 (4): 330-344. doi: 10.1080/02786829408959689
[10] Schemid H J, Stolz S, Buggisch H. On the modeling of theelectro-hydrodynamic flow field in electrostatic precipitatorsflow[J]. Flow, Turbulence and Combustion, 2002, 68: 63-89. doi: 10.1023/A:1015666116174
[11] Chun Y, Berezin N, Brocilo A A, et al. Numerical modelling of near corona wire electrohydrodynamicflow in a wire plate electrostatic precipitator[J]. IEEE Transaction on Dielectrics and Electrical Insulation, 2007, 14 (1): 119-124. doi: 10.1109/TDEI.2007.302879
[12] Launder B E, Spalding D B. The numerical computation of turbulent flows[J]. Computer Method in Applied Mechanics and Engineering, 1974(3): 269-289.
[13] White H J. Industrial electrostatic precipitation[M]. Boston: Addison Wesley, 1963.
下载:
计量
- 文章访问数: 54
- HTML全文浏览量: 25
- PDF下载量: 5
